Angles of lines and curvy parts

Posted by Tino Cogin of Samos June 05, 2022

Angles of lines and curvy parts

The Elements (Ancient Greek: Στοιχεῖα Stoikheîa) is a mathematical treatise consisting of 13 books attributed to the ancient Greek mathematician Euclid in Alexandria, Ptolemaic Egypt c. 300 BC. It is a collection of definitions, postulates, propositions (theorems and constructions), and mathematical proofs of the propositions. The books cover plane and solid Euclidean geometry, elementary number theory, and incommensurable lines. Elements is the oldest extant large-scale deductive treatment of mathematics. It has proven instrumental in the development of logic and modern science, and its logical rigor was not surpassed until the 19th century.

Euclid's Elements has been referred to as the most successful^[a]^[b] and influential^[c] textbook ever written. It was one of the very earliest mathematical works to be printed after the invention of the printing press and has been estimated to be second only to the Bible in the number of editions published since the first printing in 1482,^[1] the number reaching well over one thousand.^[d] For centuries, when the quadrivium was included in the curriculum of all university students, knowledge of at least part of Euclid's Elements was required of all students. Not until the 20th century, by which time its content was universally taught through other school textbooks, did it cease to be considered something all educated people had read...Theon of Alexandria (/ˌθiːən, -ɒn/; Ancient Greek: Θέων ὁ Ἀλεξανδρεύς; c. AD 335 – c. 405) was a Greek^[1] scholar and mathematician who lived in Alexandria, Egypt. He edited and arranged Euclid's Elements and wrote commentaries on works by Euclid and Ptolemy. His daughter Hypatia also won fame as a mathematician.

Geometry emerged as an indispensable part of the standard education of the English gentleman in the eighteenth century; by the Victorian period it was also becoming an important part of the education of artisans, children at Board Schools, colonial subjects and, to a rather lesser degree, women. The standard textbook for this purpose was none other than Euclid's The Elements.^[2]

In the 4th century AD, Theon of Alexandria produced an edition of Euclid which was so widely used that it became the only surviving source until François Peyrard's 1808 discovery at the Vatican of a manuscript not derived from Theon's. This manuscript, the Heiberg manuscript, is from a Byzantine workshop around 900 and is the basis of modern editions.^[7] Papyrus Oxyrhynchus 29 is a tiny fragment of an even older manuscript, but

only contains the statement of one proposition...

Properties[edit]

Every circle has an inscribed triangle with any three given angle measures (summing of course to 180°), and every triangle can be inscribed in some circle (which is called its circumscribed circle or circumcircle).
Every triangle has an inscribed circle, called the incircle.
Every circle has an inscribed regular polygon of n sides, for any n≥3, and every regular polygon can be inscribed in some circle (called its circumcircle).
Every regular polygon has an inscribed circle (called its incircle), and every circle can be inscribed in some regular polygon of n sides, for any n≥3.
Not every polygon with more than three sides has an inscribed circle; those polygons that do are called tangential polygons. Not every polygon with more than three sides is an inscribed polygon of a circle; those polygons that are so inscribed are called cyclic polygons.
Every triangle can be inscribed in an ellipse, called its Steiner circumellipse or simply its Steiner ellipse, whose center is the triangle's centroid.
Every triangle has an infinitude of inscribed ellipses. One of them is a circle, and one of them is the Steiner inellipse which is tangent to the triangle at the midpoints of the sides.
Every acute triangle has three inscribed squares. In a right triangle two of them are merged and coincide with each other, so there are only two distinct inscribed squares. An obtuse triangle has a single inscribed square, with one side coinciding with part of the triangle's longest side.
A Reuleaux triangle, or more generally any curve of constant width, can be inscribed with any orientation inside a square of the appropriate size.
A proof from Euclid's Elements that, given a line segment, one may construct an equilateral triangle that includes the segment as one of its sides: an equilateral triangle ΑΒΓ is made by drawing circles Δ and Ε centered on the points Α and Β, and taking one intersection of the circles as the third vertex of the triangle

Proof by contradiction
From Wikipedia, the free encyclopedia
Jump to navigation Jump to search
In logic and mathematics, proof by contradiction is a form of proof that establishes the truth or the validity of a proposition, by showing that assuming the proposition to be false leads to a contradiction. Proof by contradiction is also known as indirect proof, proof by assuming the opposite,^{[citation needed]} and reductio ad impossibile.^[1]

Law of noncontradiction
From Wikipedia, the free encyclopedia
Jump to navigation Jump to search
For the Fargo episode, see The Law of Non-Contradiction.
This article uses forms of logical notation. For a concise description of the symbols used in this notation, see List of logic symbols.
In logic, the law of non-contradiction (LNC) (also known as the law of contradiction, principle of non-contradiction (PNC), or the principle of contradiction) states that contradictory propositions cannot both be true in the same sense at the same time, e. g. the two propositions "p is the case" and "p is not the case" are mutually exclusive. Formally this is expressed as the tautology ¬(p ∧ ¬p). The law is not to be confused with the law of excluded middle which states that at least one, "p is the case" or "p is not the case" holds.
One reason to have this law is the principle of explosion, which states that anything follows from a contradiction. The law is employed in a reductio ad absurdum proof.
To express the fact that the law is tenseless and to avoid equivocation, sometimes the law is amended to say "contradictory propositions cannot both be true 'at the same time and in the same sense'".
It is one of the so called three laws of thought, along with its complement, the law of excluded middle, and the law of identity. However, no system of logic is built on just these laws, and none of these laws provide inference rules, such as modus ponens or De Morgan's laws.
The law of non-contradiction and the law of excluded middle create a dichotomy in "logical space", wherein the two parts are "mutually exclusive" and "jointly exhaustive". The law of non-contradiction is merely an expression of the mutually exclusive aspect of that dichotomy, and the law of excluded middle, an expression of its jointly exhaustive aspect.
Interpretations[edit]
One difficulty in applying the law of non-contradiction is ambiguity in the propositions.^[1] For instance, if is not explicitly specified as part of the propositions A and B, then A may be B at one time, and not at another. A and B may in some cases be made to sound mutually exclusive linguistically even though A may be partly B and partly not B at the same time. However, it is impossible to predicate of the same thing, at the same time, and in the same sense, the absence and the presence of the same fixed quality.
Heraclitus[edit]
According to both Plato and Aristotle,^[2] Heraclitus was said to have denied the law of non-contradiction. This is quite likely^[3] if, as Plato pointed out, the law of non-contradiction does not hold for changing things in the world. If a philosophy of Becoming is not possible without change, then (the potential of) what is to become must already exist in the present object. In "We step and do not step into the same rivers; we are and we are not", both Heraclitus's and Plato's object simultaneously must, in some sense, be both what it now is and have the potential (dynamic) of what it might become.^[4]
so little remains of Heraclitus' aphorisms that not much about his philosophy can be said with certainty. He seems to have held that strife of opposites is universal both within and without, therefore both opposite existents or qualities must simultaneously exist, although in some instances in different respects. "The road up and down are one and the same" implies either the road leads both ways, or there can be no road at all. This is the logical complement of the law of non-contradiction. According to Heraclitus, change, and the constant conflict of opposites is the universal logos of nature.
Protagoras[edit]
Personal subjective perceptions or judgments can only be said to be true at the same time in the same respect, in which case, the law of non-contradiction must be applicable to personal judgments. The most famous saying of Protagoras is: "Man is the measure of all things: of things which are, that they are, and of things which are not, that they are not".^[5] However, Protagoras was referring to things that are used by or in some way related to humans. This makes a great difference in the meaning of his aphorism. Properties, social entities, ideas, feelings, judgments, etc. originate in the human mind. However, Protagoras has never suggested that man must be the measure of stars or the motion of the stars.
Parmenides[edit]
Parmenides employed an ontological version of the law of non-contradiction to prove that being is and to deny the void, change, and motion. He also similarly disproved contrary propositions. In his poem On Nature, he said,
the only routes of inquiry there are for thinking:
the one that [it] is and that [it] cannot not be
is the path of Persuasion (for it attends upon truth)
the other, that [it] is not and that it is right that [it] not be,
this I point out to you is a path wholly inscrutable
for you could not know what is not (for it is not to be accomplished)
nor could you point it out… For the same thing is for thinking and for being
The nature of the ‘is’ or what-is in Parmenides is a highly contentious subject. Some have taken it to be whatever exists, some to be whatever is or can be the object of scientific inquiry.^[6]
Socrates[edit]
In Plato's early dialogues, Socrates uses the elenctic method to investigate the nature or definition of ethical concepts such as justice or virtue. Elenctic refutation depends on a dichotomous thesis, one that may be divided into exactly two mutually exclusive parts, only one of which may be true. Then Socrates goes on to demonstrate the contrary of the commonly accepted part using the law of non-contradiction. According to Gregory Vlastos,^[7] the method has the following steps:
1. Socrates' interlocutor asserts a thesis, for example, "Courage is endurance of the soul", which Socrates considers false and targets for refutation.
2. Socrates secures his interlocutor's agreement to further premises, for example, "Courage is a fine thing" and "Ignorant endurance is not a fine thing".
3. Socrates then argues, and the interlocutor agrees, that these further premises imply the contrary of the original thesis, in this case, it leads to: "courage is not endurance of the soul".
4. Socrates then claims that he has shown that his interlocutor's thesis is false and that its negation is true.
Plato's synthesis[edit]
Plato's version of the law of non-contradiction states that "The same thing clearly cannot act or be acted upon in the same part or in relation to the same thing at the same time, in contrary ways" (The Republic (436b)). In this, Plato carefully phrases three axiomatic restrictions on action or reaction: in the same part, in the same relation, at the same time. The effect is to momentarily create a frozen, timeless state, somewhat like figures frozen in action on the frieze of the Parthenon.^[8]
This way, he accomplishes two essential goals for his philosophy. First, he logically separates the Platonic world of constant change^[9] from the formally knowable world of momentarily fixed physical objects.^[10]^[11] Second, he provides the conditions for the dialectic method to be used in finding definitions, as for example in the Sophist. So Plato's law of non-contradiction is the empirically derived necessary starting point for all else he has to say.^[12]
In contrast, Aristotle reverses Plato's order of derivation. Rather than starting with experience, Aristotle begins a priori with the law of non-contradiction as the fundamental axiom of an analytic philosophical system.^[13] This axiom then necessitates the fixed, realist model. Now, he starts with much stronger logical foundations than Plato's non-contrariety of action in reaction to conflicting demands from the three parts of the soul.
Aristotle's contribution[edit]
The traditional source of the law of non-contradiction is Aristotle's Metaphysics where he gives three different versions.^[14]
- Ontological: "It is impossible that the same thing belong and not belong to the same thing at the same time and in the same respect." (1005b19-20)
- Psychological: "No one can believe that the same thing can (at the same time) be and not be." (1005b23-24)^[15]
- Logical (aka the medieval Lex Contradictoriarum):^[16] "The most certain of all basic principles is that contradictory propositions are not true simultaneously." (1011b13-14)
Aristotle attempts several proofs of this law. He first argues that every expression has a single meaning (otherwise we could not communicate with one another). This rules out the possibility that by "to be a man", "not to be a man" is meant. But "man" means "two-footed animal" (for example), and so if anything is a man, it is necessary (by virtue of the meaning of "man") that it must be a two-footed animal, and so it is impossible at the same time for it not to be a two-footed animal. Thus "it is not possible to say truly at the same time that the same thing is and is not a man" (Metaphysics 1006b 35). Another argument is that anyone who believes something cannot believe its contradiction (1008b).
Why does he not just get up first thing and walk into a well or, if he finds one, over a cliff? In fact, he seems rather careful about cliffs and wells.^[17]

Logic, Ontological Neutrality, and the Law of Non-Contradiction Achille C. Varzi Department of Philosophy, Columbia University, New York [Final version published in Elena Ficara (ed.), Contradictions. Logic, History, Actuality, Berlin: De Gruyter, 2014, pp. 53–80] Abstract. As a general theory of reasoning—and as a theory of what holds true under every possible circumstance—logic is supposed to be ontologically neutral. It ought to have nothing to do with questions concerning what there is, or whether there is anything at all. It is for this reason that traditional Aristotelian logic, with its tacit existential presuppositions, was eventually deemed inadequate as a canon of pure logic. And it is for this reason that modern quantification theory, too, with its residue of existentially loaded theorems and inferential patterns, has been claimed to suffer from a defect of logical purity. The law of non-contradiction rules out certain circumstances as impossible—circumstances in which a statement is both true and false, or perhaps circumstances where something both is and is not the case. Is this to be regarded as a further ontological bias? If so, what does it mean to forego such a bias in the interest of greater neutrality—and ought we to do so?
.
Law of the excluded middle[edit]
Main article: Law of the excluded middle
Proof by contradiction also depends on the law of the excluded middle, also first formulated by Aristotle. This states that either an assertion or its negation must be true
$\forall P\vdash (P\lor \lnot P)$
(For all propositions P, either P or not-P is true)
That is, there is no other truth value besides "true" and "false" that a proposition can take. Combined with the principle of noncontradiction, this means that exactly one of $P$ and $\lnot P$ is true. In proof by contradiction, this permits the conclusion that since the possibility of $\lnot P$ has been excluded, $P$ must be true.
Intuitionist mathematicians do not accept the law of the excluded middle, and thus reject arbitrary proof by contradiction as a viable proof technique. However, they do accept the following variation, called "proof of negation".
If the proposition to be proved has itself the form of a negation $\lnot P$ , a proof by contradiction can start by assuming that $P$ is true and derive a contradiction from that assumption. It then follows that the assumption was wrong, so $P$ is false. In such cases, the proof does not need to appeal to the law of the excluded middle.^[2] An example is the proof of irrationality of the square root of 2 given below.
History[edit]
Aristotle[edit]
The earliest known formulation is in Aristotle's discussion of the principle of non-contradiction, first proposed in On Interpretation,^[4] where he says that of two contradictory propositions (i.e. where one proposition is the negation of the other) one must be true, and the other false.^[5] He also states it as a principle in the Metaphysics book 3, saying that it is necessary in every case to affirm or deny,^[6] and that it is impossible that there should be anything between the two parts of a contradiction.^[7]
Aristotle wrote that ambiguity can arise from the use of ambiguous names, but cannot exist in the facts themselves:
It is impossible, then, that "being a man" should mean precisely "not being a man", if "man" not only signifies something about one subject but also has one significance. … And it will not be possible to be and not to be the same thing, except in virtue of an ambiguity, just as if one whom we call "man", and others were to call "not-man"; but the point in question is not this, whether the same thing can at the same time be and not be a man in name, but whether it can be in fact. (Metaphysics 4.4, W.D. Ross (trans.), GBWW 8, 525–526).
Aristotle's assertion that "it will not be possible to be and not to be the same thing", which would be written in propositional logic as ~(P ∧ ~P), is a statement modern logicians could call the law of excluded middle (P ∨ ~P), as distribution of the negation of Aristotle's assertion makes them equivalent, regardless that the former claims that no statement is both true and false, while the latter requires that any statement is either true or false.
But Aristotle also writes, "since it is impossible that contradictories should be at the same time true of the same thing, obviously contraries also cannot belong at the same time to the same thing" (Book IV, CH 6, p. 531). He then proposes that "there cannot be an intermediate between contradictories, but of one subject we must either affirm or deny any one predicate" (Book IV, CH 7, p. 531). In the context of Aristotle's traditional logic, this is a remarkably precise statement of the law of excluded middle, P ∨ ~P.
Also in On Interpretation, Aristotle seems to deny the law of excluded middle in the case of future contingents, in his discussion on the sea battle.
Leibniz[edit]
Its usual form, "Every judgment is either true or false" [footnote 9] …"(from Kolmogorov in van Heijenoort, p. 421) footnote 9: "This is Leibniz's very simple formulation (see Nouveaux Essais, IV,2)" (ibid p 421)
Bertrand Russell and Principia Mathematica[edit]
The principle was stated as a theorem of propositional logic by Russell and Whitehead in Principia Mathematica as:
$\mathbf {*2\cdot 11} .\ \ \vdash .\ p\ \vee \thicksim p$ .^[8]
So just what is "truth" and "falsehood"? At the opening PM quickly announces some definitions:
Truth-values. The "truth-value" of a proposition is truth if it is true and falsehood if it is false* [*This phrase is due to Frege] … the truth-value of "p ∨ q" is truth if the truth-value of either p or q is truth, and is falsehood otherwise … that of "~ p" is the opposite of that of p …" (p. 7-8)
This is not much help. But later, in a much deeper discussion ("Definition and systematic ambiguity of Truth and Falsehood" Chapter II part III, p. 41 ff), PM defines truth and falsehood in terms of a relationship between the "a" and the "b" and the "percipient". For example "This 'a' is 'b'" (e.g. "This 'object a' is 'red'") really means "'object a' is a sense-datum" and "'red' is a sense-datum", and they "stand in relation" to one another and in relation to "I". Thus what we really mean is: "I perceive that 'This object a is red'" and this is an undeniable-by-3rd-party "truth".
PM further defines a distinction between a "sense-datum" and a "sensation":
That is, when we judge (say) "this is red", what occurs is a relation of three terms, the mind, and "this", and "red". On the other hand, when we perceive "the redness of this", there is a relation of two terms, namely the mind and the complex object "the redness of this" (pp. 43–44).
Russell reiterated his distinction between "sense-datum" and "sensation" in his book The Problems of Philosophy (1912), published at the same time as PM (1910–1913):
Let us give the name of "sense-data" to the things that are immediately known in sensation: such things as colours, sounds, smells, hardnesses, roughnesses, and so on. We shall give the name "sensation" to the experience of being immediately aware of these things … The colour itself is a sense-datum, not a sensation. (p. 12)
Russell further described his reasoning behind his definitions of "truth" and "falsehood" in the same book (Chapter XII, Truth and Falsehood).
Consequences of the law of excluded middle in Principia Mathematica[edit]
From the law of excluded middle, formula ✸2.1 in Principia Mathematica, Whitehead and Russell derive some of the most powerful tools in the logician's argumentation toolkit. (In Principia Mathematica, formulas and propositions are identified by a leading asterisk and two numbers, such as "✸2.1".)
✸2.1 ~p ∨ p "This is the Law of excluded middle" (PM, p. 101).
The proof of ✸2.1 is roughly as follows: "primitive idea" 1.08 defines p → q = ~p ∨ q. Substituting p for q in this rule yields p → p = ~p ∨ p. Since p → p is true (this is Theorem 2.08, which is proved separately), then ~p ∨ p must be true.
✸2.11 p ∨ ~p (Permutation of the assertions is allowed by axiom 1.4)
✸2.12 p → ~(~p) (Principle of double negation, part 1: if "this rose is red" is true then it's not true that "'this rose is not-red' is true".)
✸2.13 p ∨ ~{~(~p)} (Lemma together with 2.12 used to derive 2.14)
✸2.14 ~(~p) → p (Principle of double negation, part 2)
✸2.15 (~p → q) → (~q → p) (One of the four "Principles of transposition". Similar to 1.03, 1.16 and 1.17. A very long demonstration was required here.)
✸2.16 (p → q) → (~q → ~p) (If it's true that "If this rose is red then this pig flies" then it's true that "If this pig doesn't fly then this rose isn't red.")
✸2.17 ( ~p → ~q ) → (q → p) (Another of the "Principles of transposition".)
✸2.18 (~p → p) → p (Called "The complement of reductio ad absurdum. It states that a proposition which follows from the hypothesis of its own falsehood is true" (PM, pp. 103–104).)
Most of these theorems—in particular ✸2.1, ✸2.11, and ✸2.14—are rejected by intuitionism. These tools are recast into another form that Kolmogorov cites as "Hilbert's four axioms of implication" and "Hilbert's two axioms of negation" (Kolmogorov in van Heijenoort, p. 335).
Propositions ✸2.12 and ✸2.14, "double negation": The intuitionist writings of L. E. J. Brouwer refer to what he calls "the principle of the reciprocity of the multiple species, that is, the principle that for every system the correctness of a property follows from the impossibility of the impossibility of this property" (Brouwer, ibid, p. 335).
This principle is commonly called "the principle of double negation" (PM, pp. 101–102). From the law of excluded middle (✸2.1 and ✸2.11), PM derives principle ✸2.12 immediately. We substitute ~p for p in 2.11 to yield ~p ∨ ~(~p), and by the definition of implication (i.e. 1.01 p → q = ~p ∨ q) then ~p ∨ ~(~p)= p → ~(~p). QED (The derivation of 2.14 is a bit more involved.)
Reichenbach[edit]
It is correct, at least for bivalent logic—i.e. it can be seen with a Karnaugh map—that this law removes "the middle" of the inclusive-or used in his law (3). And this is the point of Reichenbach's demonstration that some believe the exclusive-or should take the place of the inclusive-or.
About this issue (in admittedly very technical terms) Reichenbach observes:
The tertium non datur
29. (x)[f(x) ∨ ~f(x)]
is not exhaustive in its major terms and is therefore an inflated formula. This fact may perhaps explain why some people consider it unreasonable to write (29) with the inclusive-'or', and want to have it written with the sign of the exclusive-'or'
30. (x)[f(x) ⊕ ~f(x)], where the symbol "⊕" signifies exclusive-or^[9]
in which form it would be fully exhaustive and therefore nomological in the narrower sense. (Reichenbach, p. 376)
In line (30) the "(x)" means "for all" or "for every", a form used by Russell and Reichenbach; today the symbolism is usually $\forall$ x. Thus an example of the expression would look like this:
- (pig): (Flies(pig) ⊕ ~Flies(pig))
- (For all instances of "pig" seen and unseen): ("Pig does fly" or "Pig does not fly" but not both simultaneously)
Formalists versus Intuitionists[edit]
From the late 1800s through the 1930s, a bitter, persistent debate raged between Hilbert and his followers versus Hermann Weyl and L. E. J. Brouwer. Brouwer's philosophy, called intuitionism, started in earnest with Leopold Kronecker in the late 1800s.
Hilbert intensely disliked Kronecker's ideas:
Kronecker insisted that there could be no existence without construction. For him, as for Paul Gordan [another elderly mathematician], Hilbert's proof of the finiteness of the basis of the invariant system was simply not mathematics. Hilbert, on the other hand, throughout his life was to insist that if one can prove that the attributes assigned to a concept will never lead to a contradiction, the mathematical existence of the concept is thereby established (Reid p. 34)
It was his [Kronecker's] contention that nothing could be said to have mathematical existence unless it could actually be constructed with a finite number of positive integers (Reid p. 26)
The debate had a profound effect on Hilbert. Reid indicates that Hilbert's second problem (one of Hilbert's problems from the Second International Conference in Paris in 1900) evolved from this debate (italics in the original):
In his second problem, [Hilbert] had asked for a mathematical proof of the consistency of the axioms of the arithmetic of real numbers.
To show the significance of this problem, he added the following observation:
"If contradictory attributes be assigned to a concept, I say that mathematically the concept does not exist" (Reid p. 71)
Thus, Hilbert was saying: "If p and ~p are both shown to be true, then p does not exist", and was thereby invoking the law of excluded middle cast into the form of the law of contradiction.
And finally constructivists … restricted mathematics to the study of concrete operations on finite or potentially (but not actually) infinite structures; completed infinite totalities … were rejected, as were indirect proof based on the Law of Excluded Middle. Most radical among the constructivists were the intuitionists, led by the erstwhile topologist L. E. J. Brouwer (Dawson p. 49)
The rancorous debate continued through the early 1900s into the 1920s; in 1927 Brouwer complained about "polemicizing against it [intuitionism] in sneering tones" (Brouwer in van Heijenoort, p. 492). But the debate was fertile: it resulted in Principia Mathematica (1910–1913), and that work gave a precise definition to the law of excluded middle, and all this provided an intellectual setting and the tools necessary for the mathematicians of the early 20th century:
Out of the rancor, and spawned in part by it, there arose several important logical developments; Zermelo's axiomatization of set theory (1908a), that was followed two years later by the first volume of Principia Mathematica, in which Russell and Whitehead showed how, via the theory of types: much of arithmetic could be developed by logicist means (Dawson p. 49)
Brouwer reduced the debate to the use of proofs designed from "negative" or "non-existence" versus "constructive" proof:
According to Brouwer, a statement that an object exists having a given property means that, and is only proved, when a method is known which in principle at least will enable such an object to be found or constructed …
Hilbert naturally disagreed.
"pure existence proofs have been the most important landmarks in the historical development of our science," he maintained. (Reid p. 155)
Brouwer refused to accept the logical principle of the excluded middle, His argument was the following:
"Suppose that A is the statement "There exists a member of the set S having the property P." If the set is finite, it is possible—in principle—to examine each member of S and determine whether there is a member of S with the property P or that every member of S lacks the property P." (this was missing a closing quote) For finite sets, therefore, Brouwer accepted the principle of the excluded middle as valid. He refused to accept it for infinite sets because if the set S is infinite, we cannot—even in principle—examine each member of the set. If, during the course of our examination, we find a member of the set with the property P, the first alternative is substantiated; but if we never find such a member, the second alternative is still not substantiated.
Since mathematical theorems are often proved by establishing that the negation would involve us in a contradiction, this third possibility which Brouwer suggested would throw into question many of the mathematical statements currently accepted.
"Taking the Principle of the Excluded Middle from the mathematician," Hilbert said, "is the same as … prohibiting the boxer the use of his fists."
"The possible loss did not seem to bother Weyl … Brouwer's program was the coming thing, he insisted to his friends in Zürich." (Reid, p. 149)}}
In his lecture in 1941 at Yale and the subsequent paper, Gödel proposed a solution: "that the negation of a universal proposition was to be understood as asserting the existence … of a counterexample" (Dawson, p. 157))
Gödel's approach to the law of excluded middle was to assert that objections against "the use of 'impredicative definitions'" had "carried more weight" than "the law of excluded middle and related theorems of the propositional calculus" (Dawson p. 156). He proposed his "system Σ … and he concluded by mentioning several applications of his interpretation. Among them were a proof of the consistency with intuitionistic logic of the principle ~ (∀A: (A ∨ ~A)) (despite the inconsistency of the assumption ∃ A: ~ (A ∨ ~A))" (Dawson, p. 157) (no closing parenthesis had been placed)
The debate seemed to weaken: mathematicians, logicians and engineers continue to use the law of excluded middle (and double negation) in their daily work.
Intuitionist definitions of the law (principle) of excluded middle[edit]
The following highlights the deep mathematical and philosophic problem behind what it means to "know", and also helps elucidate what the "law" implies (i.e. what the law really means). Their difficulties with the law emerge: that they do not want to accept as true implications drawn from that which is unverifiable (untestable, unknowable) or from the impossible or the false. (All quotes are from van Heijenoort, italics added).
Brouwer offers his definition of "principle of excluded middle"; we see here also the issue of "testability":
On the basis of the testability just mentioned, there hold, for properties conceived within a specific finite main system, the "principle of excluded middle", that is, the principle that for every system every property is either correct [richtig] or impossible, and in particular the principle of the reciprocity of the complementary species, that is, the principle that for every system the correctness of a property follows from the impossibility of the impossibility of this property. (335)^{[citation needed]}
Kolmogorov's definition cites Hilbert's two axioms of negation
1. A → (~A → B)
2. (A → B) → { (~A → B) → B}
Hilbert's first axiom of negation, "anything follows from the false", made its appearance only with the rise of symbolic logic, as did the first axiom of implication … while … the axiom under consideration [axiom 5] asserts something about the consequences of something impossible: we have to accept B if the true judgment A is regarded as false …
Hilbert's second axiom of negation expresses the principle of excluded middle. The principle is expressed here in the form in which is it used for derivations: if B follows from A as well as from ~A, then B is true. Its usual form, "every judgment is either true or false" is equivalent to that given above".
From the first interpretation of negation, that is, the interdiction from regarding the judgment as true, it is impossible to obtain the certitude that the principle of excluded middle is true … Brouwer showed that in the case of such transfinite judgments the principle of excluded middle cannot be considered obvious
footnote 9: "This is Leibniz's very simple formulation (see Nouveaux Essais, IV,2). The formulation "A is either B or not-B" has nothing to do with the logic of judgments.
footnote 10: "Symbolically the second form is expressed thus
A ∨ ~A
where ∨ means "or". The equivalence of the two forms is easily proved (p. 421)
Examples[edit]
For example, if P is the proposition:
Socrates is mortal.
then the law of excluded middle holds that the logical disjunction:
Either Socrates is mortal, or it is not the case that Socrates is mortal.
is true by virtue of its form alone. That is, the "middle" position, that Socrates is neither mortal nor not-mortal, is excluded by logic, and therefore either the first possibility (Socrates is mortal) or its negation (it is not the case that Socrates is mortal) must be true.
An example of an argument that depends on the law of excluded middle follows.^[10] We seek to prove that
there exist two irrational numbers $a$ and $b$ such that $a^{b}$ is rational.
It is known that ${\sqrt {2}}$ is irrational (see proof). Consider the number
${\sqrt {2}}^{\sqrt {2}}$ .
Clearly (excluded middle) this number is either rational or irrational. If it is rational, the proof is complete, and
$a={\sqrt {2}}$ and $b={\sqrt {2}}$ .
But if ${\sqrt {2}}^{\sqrt {2}}$ is irrational, then let
$a={\sqrt {2}}^{\sqrt {2}}$ and $b={\sqrt {2}}$ .
Then
$a^{b}=\left({\sqrt {2}}^{\sqrt {2}}\right)^{\sqrt {2}}={\sqrt {2}}^{\left({\sqrt {2}}\cdot {\sqrt {2}}\right)}={\sqrt {2}}^{2}=2$ ,
and 2 is certainly rational. This concludes the proof.
In the above argument, the assertion "this number is either rational or irrational" invokes the law of excluded middle. An intuitionist, for example, would not accept this argument without further support for that statement. This might come in the form of a proof that the number in question is in fact irrational (or rational, as the case may be); or a finite algorithm that could determine whether the number is rational.
Non-constructive proofs over the infinite[edit]
The above proof is an example of a non-constructive proof disallowed by intuitionists:
The proof is non-constructive because it doesn't give specific numbers $a$ and $b$ that satisfy the theorem but only two separate possibilities, one of which must work. (Actually $a={\sqrt {2}}^{\sqrt {2}}$ is irrational but there is no known easy proof of that fact.) (Davis 2000:220)
(Constructive proofs of the specific example above are not hard to produce; for example $a={\sqrt {2}}$ and $b=\log _{2}9$ are both easily shown to be irrational, and $a^{b}=3$ ; a proof allowed by intuitionists).
By non-constructive Davis means that "a proof that there actually are mathematic entities satisfying certain conditions would not have to provide a method to exhibit explicitly the entities in question." (p. 85). Such proofs presume the existence of a totality that is complete, a notion disallowed by intuitionists when extended to the infinite—for them the infinite can never be completed:
In classical mathematics there occur non-constructive or indirect existence proofs, which intuitionists do not accept. For example, to prove there exists an n such that P(n), the classical mathematician may deduce a contradiction from the assumption for all n, not P(n). Under both the classical and the intuitionistic logic, by reductio ad absurdum this gives not for all n, not P(n). The classical logic allows this result to be transformed into there exists an n such that P(n), but not in general the intuitionistic … the classical meaning, that somewhere in the completed infinite totality of the natural numbers there occurs an n such that P(n), is not available to him, since he does not conceive the natural numbers as a completed totality.^[11] (Kleene 1952:49–50)
David Hilbert and Luitzen E. J. Brouwer both give examples of the law of excluded middle extended to the infinite. Hilbert's example: "the assertion that either there are only finitely many prime numbers or there are infinitely many" (quoted in Davis 2000:97); and Brouwer's: "Every mathematical species is either finite or infinite." (Brouwer 1923 in van Heijenoort 1967:336). In general, intuitionists allow the use of the law of excluded middle when it is confined to discourse over finite collections (sets), but not when it is used in discourse over infinite sets (e.g. the natural numbers). Thus intuitionists absolutely disallow the blanket assertion: "For all propositions P concerning infinite sets D: P or ~P" (Kleene 1952:48).^[12]
Putative counterexamples to the law of excluded middle include the liar paradox or Quine's paradox. Certain resolutions of these paradoxes, particularly Graham Priest's dialetheism as formalised in LP, have the law of excluded middle as a theorem, but resolve out the Liar as both true and false. In this way, the law of excluded middle is true, but because truth itself, and therefore disjunction, is not exclusive, it says next to nothing if one of the disjuncts is paradoxical, or both true and false.
Greek: τῶν δὲ τριπλεύρων σχημάτων ἰσόπλευρον μὲν τρίγωνόν ἐστι τὸ τὰς τρεῖς ἴσας ἔχον πλευράς, ἰσοσκελὲς δὲ τὸ τὰς δύο μόνας ἴσας ἔχον πλευράς, σκαληνὸν δὲ τὸ τὰς τρεῖς ἀνίσους ἔχον πλευράς, lit. 'Of trilateral figures, an isopleuron [equilateral] triangle is that which has its three sides equal, an isosceles that which has two of its sides alone equal, and a scalene that which has its three sides unequal.'^[3]
- the side lengths a, b, and c;
- the semiperimeter s = (a + b + c) / 2 (half the perimeter p);
- the angle measures A, B, and C of the angles of the vertices opposite the respective sides a, b, and c (with the vertices denoted with the same symbols as their angle measures);
- the values of trigonometric functions of the angles;
- the area T of the triangle;
- the medians m_a, m_b, and m_c of the sides (each being the length of the line segment from the midpoint of the side to the opposite vertex);
- the altitudes h_a, h_b, and h_c (each being the length of a segment perpendicular to one side and reaching from that side (or possibly the extension of that side) to the opposite vertex);
- the lengths of the internal angle bisectors t_a, t_b, and t_c (each being a segment from a vertex to the opposite side and bisecting the vertex's angle);
- the perpendicular bisectors p_a, p_b, and p_c of the sides (each being the length of a segment perpendicular to one side at its midpoint and reaching to one of the other sides);
- the lengths of line segments with an endpoint at an arbitrary point P in the plane (for example, the length of the segment from P to vertex A is denoted PA or AP);
- the inradius r (radius of the circle inscribed in the triangle, tangent to all three sides), the exradii r_a, r_b, and r_c (each being the radius of an excircle tangent to side a, b, or c respectively and tangent to the extensions of the other two sides), and the circumradius R (radius of the circle circumscribed around the triangle and passing through all three vertices).
- The theorem is used in triangulation, for solving a triangle or circle, i.e., to find (see Figure 3):
  - the third side of a triangle if one knows two sides and the angle between them: $c={\sqrt {a^{2}+b^{2}-2ab\cos \gamma }}\,;$
  - the angles of a triangle if one knows the three sides: $\gamma =\arccos \left({\frac {a^{2}+b^{2}-c^{2}}{2ab}}\right)\,;$
  - the third side of a triangle if one knows two sides and an angle opposite to one of them (one may also use the Pythagorean theorem to do this if it is a right triangle):
    $a=b\cos \gamma \pm {\sqrt {c^{2}-b^{2}\sin ^{2}\gamma }}\,.$
    $a=b\cos \gamma \pm {\sqrt {c^{2}-b^{2}\sin ^{2}\gamma }}\,.$ The triangle inequality provides two more interesting constraints for triangles whose sides are a, b, c, where a ≥ b ≥ c and $\phi$ is the golden ratio, as
    $1<{\frac {a+c}{b}}<3$
    $1\leq \min \left({\frac {a}{b}},{\frac {b}{c}}\right)<\phi .$ ^[8]
    ^{Reverse triangle inequality[edit]
    The reverse triangle inequality is an elementary consequence of the triangle inequality that gives lower bounds instead of upper bounds. For plane geometry, the statement is:^[19]
    Any side of a triangle is greater than or equal to the difference between the other two sides.
    In the case of a normed vector space, the statement is:
    ${\bigg |}\|x\|-\|y\|{\bigg |}\leq \|x-y\|,$
    or for metric spaces, $| d (y, x) - d (x, z)| \leq d (y, z)$ . This implies that the norm $\|\cdot \|$ as well as the distance function $d(x,\cdot )$ are Lipschitz continuous with Lipschitz constant $1$ , and therefore are in particular uniformly continuous.
    The proof for the reverse triangle uses the regular triangle inequality, and $\|y-x\|=\|{-}1(x-y)\|=|{-}1|\cdot \|x-y\|=\|x-y\|$ :
    $\|x\|=\|(x-y)+y\|\leq \|x-y\|+\|y\|\Rightarrow \|x\|-\|y\|\leq \|x-y\|,$
    $\|y\|=\|(y-x)+x\|\leq \|y-x\|+\|x\|\Rightarrow \|x\|-\|y\|\geq -\|x-y\|,$
    Combining these two statements gives:
    $-\|x-y\|\leq \|x\|-\|y\|\leq \|x-y\|\Rightarrow {\bigg |}\|x\|-\|y\|{\bigg |}\leq \|x-y\|.$}
    ^{In a Euclidean space, the sum of angles of a triangle equals the straight angle (180 degrees, $π$ radians, two right angles, or a half-turn). A triangle has three angles, one at each vertex, bounded by a pair of adjacent sides.
    It was unknown for a long time whether other geometries exist, for which this sum is different. The influence of this problem on mathematics was particularly strong during the 19th century. Ultimately, the answer was proven to be positive: in other spaces (geometries) this sum can be greater or lesser, but it then must depend on the triangle. Its difference from 180° is a case of angular defect and serves as an important distinction}
    for geometric systems.
    Definition
    One radian is defined as the angle subtended from the center of a circle which intercepts an arc equal in length to the radius of the circle.^[3] More generally, the magnitude in radians of a subtended angle is equal to the ratio of the arc length to the radius of the circle; that is, $θ = s / r$ , where $θ$ is the subtended angle in radians, $s$ is arc length, and $r$ is radius. A right angle is exactly $π$ /2 radians.^[4]
    A complete revolution is 2 $π$ radians (shown here with a circle of radius one and thus circumference 2 $π$ ).
    The magnitude in radians of one complete revolution (360 degrees) is the length of the entire circumference divided by the radius, or $2 π r / r$ , or 2 $π$ . Thus 2 $π$ radians is equal to 360 degrees, meaning that one radian is equal to 180/ $π$ degrees ≈ 57.295779513082320876 degrees.^[5]
    The relation $2 π rad = 360°$ can be derived using the formula for arc length, $\ell _{\text{arc}}=2\pi r\left({\tfrac {\theta }{360^{\circ }}}\right)$ , and by using a circle of radius 1. Since radian is the measure of an angle that subtends an arc of a length equal to the radius of the circle, $1=2\pi \left({\tfrac {1{\text{ rad}}}{360^{\circ }}}\right)$ . This can be further simplified to $1={\tfrac {2\pi {\text{ rad}}}{360^{\circ }}}$ . Multiplying both sides by 360° gives $360° = 2 π rad$ .
    
    What is equal and not equal famous question answered by the shhhers
    operator = 180/d
    360 = 6.28318
    In circles[edit]
    Main article: Circle § Chord
    Among properties of chords of a circle are the following:
    Chords are equidistant from the center if and only if their lengths are equal.
    Equal chords are subtended by equal angles from the center of the circle.
    A chord that passes through the center of a circle is called a diameter and is the longest chord of that specific circle.
    If the line extensions (secant lines) of chords AB and CD intersect at a point P, then their lengths satisfy AP·PB = CP·PD (power of a point theorem).
    In ellipses[edit]
    The midpoints of a set of parallel chords of an ellipse are collinear.^[1]
    In trigonometry[edit]
    Chords were used extensively in the early development of trigonometry. The first known trigonometric table, compiled by Hipparchus, tabulated the value of the chord function for every 7+1/2 degrees. In the second century AD, Ptolemy of Alexandria compiled a more extensive table of chords in his book on astronomy, giving the value of the chord for angles ranging from 1/2 to 180 degrees by increments of 1/2 degree. The circle was of diameter 120, and the chord lengths are accurate to two base-60 digits after the integer part.^[2]
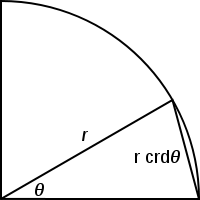
    The chord function is defined geometrically as shown in the picture. The chord of an angle is the length of the chord between two points on a unit circle separated by that central angle. The angle θ is taken in the positive sense and must lie in the interval $0 < θ \leq π$ (radian measure). The chord function can be related to the modern sine function, by taking one of the points to be (1,0), and the other point to be ( $cos θ, sin θ$ ), and then using the Pythagorean theorem to calculate the chord length:^[2]This is the average number of sixtieths of a unit that must be added to chord(θ°) each time the angle increases by one minute of arc, between the entry for θ° and that for (θ +
    1/2)°. Thus, it is used for linear interpolation. Glowatzki and Göttsche showed that Ptolemy must have calculated chords to five sexigesimal places in order to achieve the degree of accuracy found in the "sixtieths" column.^[3]
    ${\begin{array}{|l|rrr|rrr|}\hline {\text{arc}}^{\circ }&{\text{chord}}&&&{\text{sixtieths}}&&\\\hline {}\,\,\,\,\,\,\,\,\,\,{\tfrac {1}{2}}&0&31&25&0\quad 1&2&50\\{}\,\,\,\,\,\,\,1&1&2&50&0\quad 1&2&50\\{}\,\,\,\,\,\,\,1{\tfrac {1}{2}}&1&34&15&0\quad 1&2&50\\{}\,\,\,\,\,\,\,\vdots &\vdots &\vdots &\vdots &\vdots &\vdots &\vdots \\109&97&41&38&0\quad 0&36&23\\109{\tfrac {1}{2}}&97&59&49&0\quad 0&36&9\\110&98&17&54&0\quad 0&35&56\\110{\tfrac {1}{2}}&98&35&52&0\quad 0&35&42\\111&98&53&43&0\quad 0&35&29\\111{\tfrac {1}{2}}&99&11&27&0\quad 0&35&15\\112&99&29&5&0\quad 0&35&1\\112{\tfrac {1}{2}}&99&46&35&0\quad 0&34&48\\113&100&3&59&0\quad 0&34&34\\{}\,\,\,\,\,\,\,\vdots &\vdots &\vdots &\vdots &\vdots &\vdots &\vdots \\179&119&59&44&0\quad 0&0&25\\179{\frac {1}{2}}&119&59&56&0\quad 0&0&9\\180&120&0&0&0\quad 0&0&0\\\hline \end{array}}$