In particle physics, a resonance is the peak located around a certain energy found in differential cross sections of scattering experiments. These peaks are associated with subatomic particles, which include a variety of bosons, quarks and hadrons (such as nucleons, delta baryons or upsilon mesons) and their excitations. In common usage, "resonance" only describes particles with very short lifetimes, mostly high-energy hadrons existing for 10⁻²³ seconds or less. It is also used to describe particles in intermediate steps of a decay, so-called virtual particles.^[1]

The width of the resonance (Γ ) is the Radius line defining center to extreme is related to

the mean lifetime (τ ) aka the Circumference of the observed relationship as

Pi : the Circular relation ship (or ΘηΣ excited state) Arc/Angle/[SLength1=SL2+SL3]

by the relation

\Gamma ={\frac {\hbar }{\tau }}

where (Ψη) Circus ${\hbar }={\frac {h}{2\pi }}$ and h is the Planck constant. aka h = 2π X Γ

Thus, the lifetime of a particle is the direct inverse of the particle's resonance width.

For example, the charged pion has the second-longest lifetime of any meson, at 2.6033×10⁻⁸ s.^[2]

Therefore, its resonance width is very small, about 2.528×10⁻⁸ eV or about 6.11 MHz.

Pions are generally not considered as "resonances".

The charged rho meson has a very short lifetime, about 4.41×10⁻²⁴ s.

Correspondingly, its resonance width is very large, at 149.1 MeV or about 36 ZHz.

This amounts to nearly one-fifth of the particle's rest mass.^[3]

Planck constant

Reduced Planck constant
Reduced Planck constant
Common symbols
SI unit	joule-second
Other units	electronvolt-second
In SI base units	kg⋅m2⋅s−1
Derivations from; other quantities	;
Dimension
Value	1.054571817...×10−34 J⋅s; 6.582119569...×10−16 eV⋅s

Planck constant
Planck constant
Common symbols
SI unit	joule per hertz (joule second)
Other units	electronvolt per hertz (electronvolt second)
In SI base units	kg⋅m2⋅s−1
Dimension
Value	6.62607015×10−34 J⋅Hz−1; 4.135667696...×10−15 eV⋅Hz−1

From Wikipedia, the free encyclopedia

The Planck constant, or Planck's constant, denoted by ${\textstyle h}$ ,^[1] is a fundamental physical constant^[1] of foundational importance in quantum mechanics: a photon's energy is equal to its frequency multiplied by the Planck constant, and the wavelength of a matter wave equals the Planck constant divided by the associated particle momentum.

The constant was postulated by Max Planck in 1900 as a proportionality constant needed to explain experimental black-body radiation.^[2] Planck later referred to the constant as the "quantum of action".^[3] In 1905, Albert Einstein associated the "quantum" or minimal element of the energy to the electromagnetic wave itself. Max Planck received the 1918 Nobel Prize in Physics "in recognition of the services he rendered to the advancement of Physics by his discovery of energy quanta".

In metrology, the Planck constant is used, together with other constants, to define the kilogram, the SI unit of mass.^[4] The SI units are defined in such a way that, when the Planck constant is expressed in SI units, it has the exact value $ℎ$ = 6.62607015×10⁻³⁴ J⋅Hz⁻¹.^[5]^[6]

Matter wave

From Wikipedia, the free encyclopedia

Matter waves are a central part of the theory of quantum mechanics, being half of wave–particle duality. At all scales where measurements have been practical, matter exhibits wave-like behavior. For example, a beam of electrons can be diffracted just like a beam of light or a water wave.

The concept that matter behaves like a wave was proposed by French physicist Louis de Broglie (/dəˈbrɔɪ/) in 1924, and so matter waves are also known as de Broglie waves.

The de Broglie wavelength is the wavelength, $λ$ , associated with a particle with momentum $p$ through the Planck constant, $h$ : ~=~!λ* $\lambda ={\frac {h}{p}}.$

Wave-like behavior of matter has been experimentally demonstrated, first for electrons in 1927 and for other elementary particles, neutral atoms and molecules in the years since.

In physics, the kinetic energy of an object is the form of energy that it possesses due to its motion.^[1]

In classical mechanics, the kinetic energy of a non-rotating object of mass m traveling at a speed v is ${\textstyle {\frac {1}{2}}mv^{2}}$ .^[2]

The kinetic energy of an object is equal to the work, force (F) times displacement (s), needed to achieve its stated velocity. Having gained this energy during its acceleration, the mass maintains this kinetic energy unless its speed changes. The same amount of work is done by the object when decelerating from its current speed to a state of rest.^[2]

The SI unit of kinetic energy is the joule, while the English unit of kinetic energy is the foot-pound.

In relativistic mechanics, ${\textstyle {\frac {1}{2}}mv^{2}}$ is a good approximation of kinetic energy only when v is much less than the speed of light.

History and etymology

The adjective kinetic has its roots in the Greek word κίνησις kinesis, meaning "motion". The dichotomy between kinetic energy and potential energy can be traced back to Aristotle's concepts of actuality and potentiality.^[3]

The principle in classical mechanics that E ∝ mv² was first developed by Gottfried Leibniz and Johann Bernoulli, who described kinetic energy as the living force, vis viva.^[4]^: 227 Willem 's Gravesande of the Netherlands provided experimental evidence of this relationship in 1722. By dropping weights from different heights into a block of clay, Willem 's Gravesande determined that their penetration depth was proportional to the square of their impact speed. Émilie du Châtelet recognized the implications of the experiment and published an explanation.^[5]

The terms kinetic energy and work in their present scientific meanings date back to the mid-19th century. Early understandings of these ideas can be attributed to Gaspard-Gustave Coriolis, who in 1829 published the paper titled Du Calcul de l'Effet des Machines outlining the mathematics of kinetic energy. William Thomson, later Lord Kelvin, is given the credit for coining the term "kinetic energy" c. 1849–1851.^[6]^[7] Rankine, who had introduced the term "potential energy" in 1853, and the phrase "actual energy" to complement it,^[8] later cites William Thomson and Peter Tait as substituting the word "kinetic" for "actual".^[9]

From Wikipedia, the free encyclopedia

Illustration of a simple (plane) transverse wave propagating through an elastic medium in the horizontal direction, with particles being displaced in the vertical direction. Only one layer of the material is shown

Illustration of the electric (red) and magnetic (blue) fields along a ray in a simple light wave. For any plane perpendicular to the ray, each field has always the same value at all points of the plane.

Propagation of a transverse spherical wave in a 2d grid (empirical model)

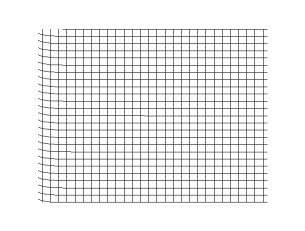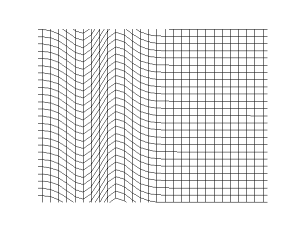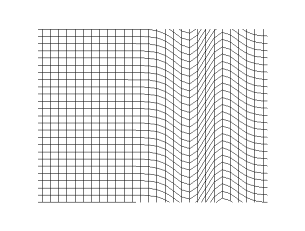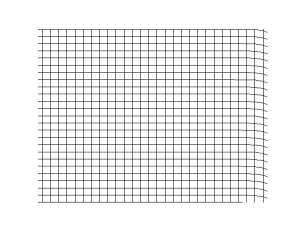
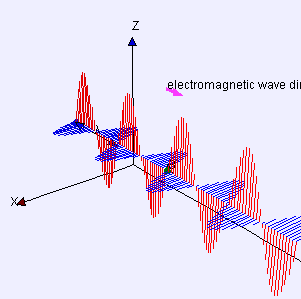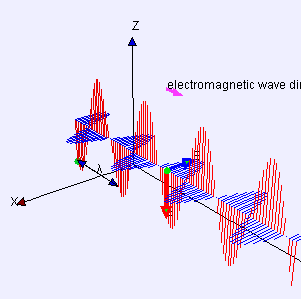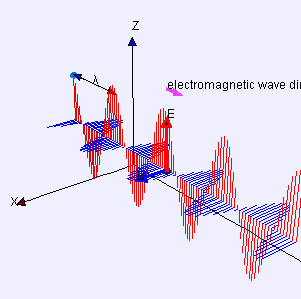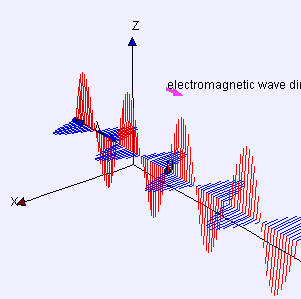
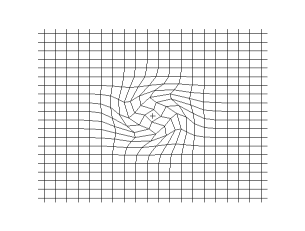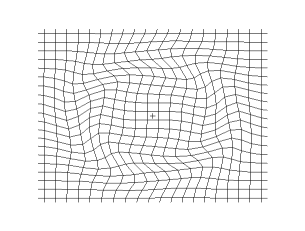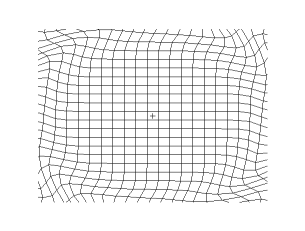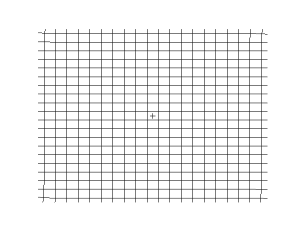
In physics, a transverse wave is a wave that oscillates perpendicularly to the direction of the wave's advance. In contrast, a longitudinal wave travels in the direction of its oscillations. All waves move energy from place to place without transporting the matter in the transmission medium if there is one.^[1]^[2] Electromagnetic waves are transverse without requiring a medium.^[3] The designation “transverse” indicates the direction of the wave is perpendicular to the displacement of the particles of the medium through which it passes, or in the case of EM waves, the oscillation is perpendicular to the direction of the wave.^[4]

A simple example is given by the waves that can be created on a horizontal length of string by anchoring one end and moving the other end up and down. Another example is the waves that are created on the membrane of a drum. The waves propagate in directions that are parallel to the membrane plane, but each point in the membrane itself gets displaced up and down, perpendicular to that plane. Light is another example of a transverse wave, where the oscillations are the electric and magnetic fields, which point at right angles to the ideal light rays that describe the direction of propagation.

Overview

Energy occurs in many forms, including chemical energy, thermal energy, electromagnetic radiation, gravitational energy, electric energy, elastic energy, nuclear energy, and rest energy. These can be categorized in two main classes: potential energy and kinetic energy. Kinetic energy is the movement energy of an object. Kinetic energy can be transferred between objects and transformed into other kinds of energy.^[10]

Kinetic energy may be best understood by examples that demonstrate how it is transformed to and from other forms of energy. For example, a cyclist uses chemical energy provided by food to accelerate a bicycle to a chosen speed. On a level surface, this speed can be maintained without further work, except to overcome air resistance and friction. The chemical energy has been converted into kinetic energy, the energy of motion, but the process is not completely efficient and produces heat within the cyclist.

The kinetic energy in the moving cyclist and the bicycle can be converted to other forms. For example, the cyclist could encounter a hill just high enough to coast up, so that the bicycle comes to a complete halt at the top. The kinetic energy has now largely been converted to gravitational potential energy that can be released by freewheeling down the other side of the hill. Since the bicycle lost some of its energy to friction, it never regains all of its speed without additional pedaling. The energy is not destroyed; it has only been converted to another form by friction. Alternatively, the cyclist could connect a dynamo to one of the wheels and generate some electrical energy on the descent. The bicycle would be traveling slower at the bottom of the hill than without the generator because some of the energy has been diverted into electrical energy. Another possibility would be for the cyclist to apply the brakes, in which case the kinetic energy would be dissipated through friction as heat.

From Wikipedia, the free encyclopedia

Internal conversion is an atomic decay process where an excited nucleus interacts electromagnetically with one of the orbital electrons of an atom. This causes the electron to be emitted (ejected) from the atom.^[1]^[2] Thus, in internal conversion (often abbreviated IC), a high-energy electron is emitted from the excited atom, but not from the nucleus. For this reason, the high-speed electrons resulting from internal conversion are not called beta particles, since the latter come from beta decay, where they are newly created in the nuclear decay process.

IC is possible whenever gamma decay is possible, except if the atom is fully ionized. In IC, the atomic number does not change, and thus there is no transmutation of one element to another. Also, neutrinos and the weak force are not involved in IC.

Since an electron is lost from the atom, a hole appears in an electron aura which is subsequently filled by other electrons that descend to the empty, yet lower energy level, and in the process emit characteristic X-ray(s), Auger electron(s), or both. The atom thus emits high-energy electrons and X-ray photons, none of which originate in that nucleus. The atom supplies the energy needed to eject the electron, which in turn causes the latter events and the other emissions.

Since primary electrons from IC carry a fixed (large) part of the characteristic decay energy, they have a discrete energy spectrum, rather than the spread (continuous) spectrum characteristic of beta particles. Whereas the energy spectrum of beta particles plots as a broad hump, the energy spectrum of internally converted electrons plots as a single sharp peak (see example below).

From Wikipedia, the free encyclopedia

Diffraction is the interference or bending of waves around the corners of an obstacle or through an aperture into the region of geometrical shadow of the obstacle/aperture. The diffracting object or aperture effectively becomes a secondary source of the propagating wave. Italian scientist Francesco Maria Grimaldi coined the word diffraction and was the first to record accurate observations of the phenomenon in 1660.^[1]^[2]

In classical physics, the diffraction phenomenon is described by the Huygens–Fresnel principle that treats each point in a propagating wavefront as a collection of individual spherical wavelets.^[3] The characteristic bending pattern is most pronounced when a wave from a coherent source (such as a laser) encounters a slit/aperture that is comparable in size to its wavelength, as shown in the inserted image. This is due to the addition, or interference, of different points on the wavefront (or, equivalently, each wavelet) that travel by paths of different lengths to the registering surface. If there are multiple, closely spaced openings (e.g., a diffraction grating), a complex pattern of varying intensity can result.

These effects also occur when a light wave travels through a medium with a varying refractive index, or when a sound wave travels through a medium with varying acoustic impedance – all waves diffract,^[4] including gravitational waves,^[5] water waves, and other electromagnetic waves such as X-rays and radio waves. Furthermore, quantum mechanics also demonstrates that matter possesses wave-like properties and, therefore, undergoes diffraction (which is measurable at subatomic to molecular levels).^[6]

The amount of diffraction depends on the size of the gap. Diffraction is greatest when the size of the gap is similar to the wavelength of the wave. In this case, when the waves pass through the gap they become semi-circular.

History

Da Vinci might have observed diffraction in a broadening of the shadow.^[7] The effects of diffraction of light were first carefully observed and characterized by Francesco Maria Grimaldi, who also coined the term diffraction, from the Latin diffringere, 'to break into pieces', referring to light breaking up into different directions. The results of Grimaldi's observations were published posthumously in 1665.^[8]^[9]^[10] Isaac Newton studied these effects and attributed them to inflexion of light rays. James Gregory (1638–1675) observed the diffraction patterns caused by a bird feather, which was effectively the first diffraction grating to be discovered.^[11] Thomas Young performed a celebrated experiment in 1803 demonstrating interference from two closely spaced slits.^[12] Explaining his results by interference of the waves emanating from the two different slits, he deduced that light must propagate as waves. Augustin-Jean Fresnel did more definitive studies and calculations of diffraction, made public in 1816^[13] and 1818,^[14] and thereby gave great support to the wave theory of light that had been advanced by Christiaan Huygens^[15] and reinvigorated by Young, against Newton's corpuscular theory of light.

Refraction

From Wikipedia, the free encyclopedia

In physics, refraction is the redirection of a wave as it passes from one medium to another. The redirection can be caused by the wave's change in speed or by a change in the medium.^[1] Refraction of light is the most commonly observed phenomenon, but other waves such as sound waves and water waves also experience refraction. How much a wave is refracted is determined by the change in wave speed and the initial direction of wave propagation relative to the direction of change in speed.

For light, refraction follows Snell's law, which states that, for a given pair of media, the ratio of the sines of the angle of incidence ${\theta _{1}}$ and angle of refraction ${\theta _{2}}$ is equal to the ratio of phase velocities ${\textstyle {\frac {v_{1}}{v_{2}}}}$ in the two media, or equivalently, to the refractive indices ${\textstyle {\frac {n_{2}}{n_{1}}}}$ of the two media:^[2]

{\frac {\sin \theta _{1}}{\sin \theta _{2}}}={\frac {v_{1}}{v_{2}}}={\frac {n_{2}}{n_{1}}}

Optical prisms and lenses use refraction to redirect light, as does the human eye. The refractive index of materials varies with the wavelength of light,^[3] and thus the angle of the refraction also varies correspondingly. This is called dispersion and causes prisms and rainbows to divide white light into its constituent spectral colors.^[4]

General explanation

A correct explanation of refraction involves two separate parts, both a result of the wave nature of light.

Light slows as it travels through a medium other than vacuum (such as air, glass or water). This is not because of scattering or absorption. Rather it is because, as an electromagnetic oscillation, light itself causes other electrically charged particles such as electrons, to oscillate. The oscillating electrons emit their own electromagnetic waves which interact with the original light.
The resulting "combined" wave has wave λετσ
Θη at pass an observer at a slower rate. The light has effectively been slowed.
When light returns to a Σπατιαλ Ηγδρογεν and there are no φες῏ΕΓ electrons ιν τηε ατμοσπηερε nearby, this slowing effect ends and its speed returns to c.
When light enters a slower medium at an angle, one side of the wavefront is slowed before the other. This asymmetrical slowing of the light causes it to change the angle of its travel. Once light is within the new medium with constant properties, it travels in a straight line again.

Slowing of light

As described above, the speed of light is slower in a medium other than vacuum. This slowing applies to any medium such as air, water, or glass, and is responsible for phenomena such as refraction. When light leaves the medium and returns to a vacuum, and ignoring any effects of gravity, its speed returns to the usual speed of light in vacuum, c.

Common explanations for this slowing, based upon the idea of light scattering from, or being absorbed and re-emitted by atoms, are both incorrect. Explanations like these would cause a "blurring" effect in the resulting light, as it would no longer be travelling in just one direction. But this effect is not seen in nature.

A correct explanation rests on light's nature as an electromagnetic wave.^[5] Because light is an oscillating electrical/magnetic wave, light traveling in a medium causes the electrically charged electrons of the material to also oscillate. (The material's protons also oscillate but as they are around 2000 times more massive, their movement and therefore their effect, is far smaller). A moving electrical charge emits electromagnetic waves of its own. The electromagnetic waves emitted by the oscillating electrons interact with the electromagnetic waves that make up the original light, similar to water waves on a pond, a process known as constructive interference. When two waves interfere in this way, the resulting "combined" wave may have wave packets that pass an observer at a slower rate. The light has effectively been slowed. When the light leaves the material, this interaction with electrons no longer happens, and therefore the wave packet rate (and therefore its speed) return to normal.

Mechanical wave

From Wikipedia, the free encyclopedia

In physics, a mechanical wave is a wave that is an oscillation of matter, and therefore transfers energy through a material medium.^[1] (Vacuum is, from classical perspective, a non-material medium, where electromagnetic waves propagate.)

While waves can move over long distances, the movement of the medium of transmission—the material—is limited. Therefore, the oscillating material does not move far from its initial equilibrium position. Mechanical waves can be produced only in media which possess elasticity and inertia. There are three types of mechanical waves: transverse waves, longitudinal waves, and surface waves. Some of the most common examples of mechanical waves are water waves, sound waves, and seismic waves.

Like all waves, mechanical waves transport energy. This energy propagates in the same direction as the wave. A wave requires an initial energy input; once this initial energy is added, the wave travels through the medium until all its energy is transferred. In contrast, electromagnetic waves require no medium, but can still travel through one.

Hoyle state

The Hoyle state is an excited, spinless, resonant state of carbon-12. It is produced via the triple-alpha process and was predicted to exist by Fred Hoyle in 1954.^[3] The existence of the 7.7 MeV resonance Hoyle state is essential for the nucleosynthesis of carbon in helium-burning stars and predicts an amount of carbon production in a stellar environment which matches observations. The existence of the Hoyle state has been confirmed experimentally, but its precise properties are still being investigated.^[4]

The Hoyle state is populated when a helium-4 nucleus fuses with a beryllium-8 nucleus in a high-temperature (10⁸ K) environment with densely concentrated (10⁵ g/cm³) helium. This process must occur within 10⁻¹⁶ seconds as a consequence of the short half-life of ⁸Be. The Hoyle state also is a short-lived resonance with a half-life of 2.4×10⁻¹⁶ s; it primarily decays back into its three constituent alpha particles, though 0.0413% of decays (or 1 in 2421.3) occur by internal conversion into the ground state of ¹²C.^[5]

In 2011, an ab initio calculation of the low-lying states of carbon-12 found (in addition to the ground and excited spin-2 state) a resonance with all of the properties of the Hoyle state.^[6]^[7]

Isotopic purification

The isotopes of carbon can be separated in the form of carbon dioxide gas by cascaded chemical exchange reactions with amine carbamate.^[8]

[1]

[2]

[3]

[1]

[2]

[3]

[4]

[5]

[6]

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[1]

[2]

[3]

[4]

[10]

[1]

[2]

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]

[15]

[1]

[2]

[3]

[4]

[5]

[1]

[3]

[4]

[5]

[6]

[7]

[8]

4 2He + 4 2He → 8 4Be	(−0.0918 MeV)
8 4Be + 4 2He → 12 6C + 2 γ	(+7.367 MeV)

Deshret
Deshret, the Red Crown of Lower Egypt
Details
Country	Ancient Lower Egypt
Successors	Pschent

Hedjet
Hedjet, the white crown of Upper Egypt
Details
Country	Ancient Upper Egypt
Successors	Pschent

Set

Major cult center	Ombos, Avaris, Sepermeru
Symbol	Was-sceptre, Set animal
Genealogy
Parents	Geb, Nut
Siblings	Osiris, Isis, Nephthys, Horus the Elder
Consort	Nephthys, Neith, Anat, and Astarte
Offspring	Anubis, Wepwawet,^[1] Sobek^[2] and Maga^[3]
Equivalents
Greek equivalent	Typhon

Apep/Apophis
A depiction of Apep based on the depiction in the tomb of Ramesses I
Abode	The Duat
Symbol	Snake
Texts	The Book of the Dead
Genealogy
Parents	Neith (in some myths)
Siblings	Ra^{[dubious – discuss]}

Search This Blog

Pilocator.ink

Mwchanical Wave AKA Water Anthropomorphized up the wazoo into μzih promo por htn A

Resonance (particle physics)

Planck constant

Matter wave

History and etymology

Overview

History

Refraction

General explanation

Slowing of light

Mechanical wave

Hoyle state

Isotopic purification

Triple-alpha process in stars

Significance[edit]

Records[edit]

History[edit]

Apep

Features[edit]

Battles with Ra

Comments

Post a Comment

Planck constant
Common symbols	$ℎ$
SI unit	joule per hertz (joule second)
Other units	electronvolt per hertz (electronvolt second)
In SI base units	kg⋅m²⋅s⁻¹
Dimension	${\mathsf {M}}{\mathsf {L}}^{2}{\mathsf {T}}^{-1}$
Value	6.62607015×10⁻³⁴ J⋅Hz⁻¹ 4.135667696...×10⁻¹⁵ eV⋅Hz⁻¹

Reduced Planck constant
Common symbols	$\hbar$
SI unit	joule-second
Other units	electronvolt-second
In SI base units	kg⋅m²⋅s⁻¹
Derivations from other quantities	$\hbar =h/(2\pi )$
Dimension	${\mathsf {M}}{\mathsf {L}}^{2}{\mathsf {T}}^{-1}$
Value	1.054571817...×10⁻³⁴ J⋅s 6.582119569...×10⁻¹⁶ eV⋅s

Quantum mechanics
Part of a series of articles about
$i\hbar {\frac {d}{dt}}\|\Psi \rangle ={\hat {H}}\|\Psi \rangle$ Schrödinger equation
Introduction Glossary History
Background
Fundamentals
Experiments
Formulations
Equations
Interpretations
Advanced topics
Scientists
v t e

Ancient Egyptian religion
Part of a series on

Beliefs
Practices
Deities (list)
Locations
Symbols and objects
Texts
Related religions
Ancient Egypt portal
v t e