He He the three mind problem with points

Three-body problem

41 languages

• Article
• Talk

• Read
• Edit
• View history

Tools

From Wikipedia, the free encyclopedia

(Redirected from 3-body problem)

This article is about the physics theory. For other uses, see Three-body problem (disambiguation).

Approximate trajectories of three identical bodies located at the vertices of a scalene triangle and having zero initial velocities. The center of mass, in accordance with the law of conservation of momentum, remains in place.

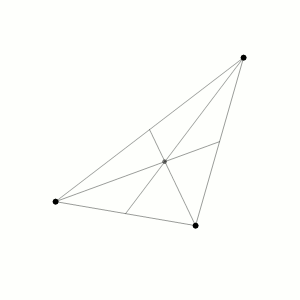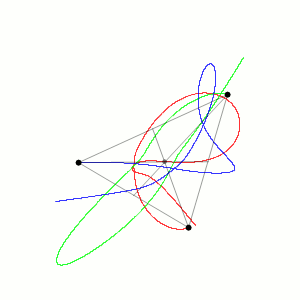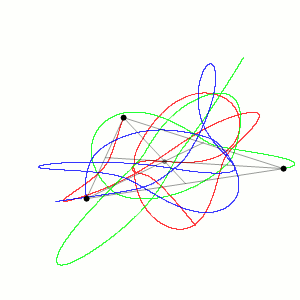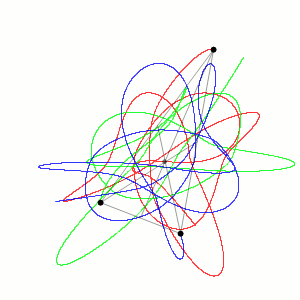
In physics, specifically classical mechanics, the three-body problem involves taking the initial positions and velocities (or momenta) of three point masses that orbit each other in space and calculating their subsequent trajectories using Newton's laws of motion and Newton's law of universal gravitation.^[1]

Unlike the two-body problem, the three-body problem has no general closed-form solution.^[1] When three bodies orbit each other, the resulting dynamical system is chaotic for most initial conditions, and the only way to predict the motions of the bodies is to calculate them using numerical methods.

The three-body problem is a special case of the n-body problem. Historically, the first specific three-body problem to receive extended study was the one involving the Moon, Earth, and the Sun.^[2] In an extended modern sense, a three-body problem is any problem in classical mechanics or quantum mechanics that models the motion of three particles.

Helium atom

9 languages

• Article
• Talk

• Read
• Edit
• View history

Tools

From Wikipedia, the free encyclopedia

This article is about the physics of atomic helium. For other properties of helium, see Helium.

Helium atom

Helium-4
Names
Systematic IUPAC name Helium[1]
Identifiers
CAS Number	7440-59-7
3D model (JSmol)	Interactive image
ChEBI	CHEBI:33681
ChemSpider	22423
EC Number	231-168-5
Gmelin Reference	16294
KEGG	D04420
MeSH	Helium
PubChem CID	23987
RTECS number	MH6520000
UNII	206GF3GB41
UN number	1046
InChI
SMILES
Properties
Chemical formula	He
Molar mass	4.002602 g·mol−1
Appearance	Colourless gas
Boiling point	−269 °C (−452.20 °F; 4.15 K)
Thermochemistry
Std molar entropy (S⦵298)	126.151-126.155 J K−1 mol−1
Pharmacology
ATC code	V03AN03 (WHO)
Except where otherwise noted, data are given for materials in their standard state (at 25 °C [77 °F], 100 kPa).  verify (what is  ?) Infobox references

A helium atom is an atom of the chemical element helium. Helium is composed of two electrons bound by the electromagnetic force to a nucleus containing two protons along with two neutrons, depending on the isotope, held together by the strong force. Unlike for hydrogen, a closed-form solution to the Schrödinger equation for the helium atom has not been found. However, various approximations, such as the Hartree–Fock method, can be used to estimate the ground state energy and wavefunction of the atom. Historically, the first such helium spectrum calculation was done by Albrecht Unsöld in 1927.^[2] Its success was considered to be one of the earliest signs of validity of Schrödinger's wave mechanics.^[3]

Comparison of classical and quantum harmonic oscillator conceptions for a single spinless particle. The two processes differ greatly. The classical process (A–B) is represented as the motion of a particle along a trajectory. The quantum process (C–H) has no such trajectory. Rather, it is represented as a wave; here, the vertical axis shows the real part (blue) and imaginary part (red) of the wave function. Panels (C–F) show four different standing-wave solutions of the Schrödinger equation. Panels (G–H) further show two different wave functions that are solutions of the Schrödinger equation but not standing waves.

The wave function of an initially very localized free particle.

In quantum physics, a wave function (or wavefunction) is a mathematical description of the quantum state of an isolated quantum system. The most common symbols for a wave function are the Greek letters ψ and Ψ (lower-case and capital psi, respectively). Wave functions are complex-valued. For example, a wave function might assign a complex number to each point in a region of space. The Born rule^[1][2][3] provides the means to turn these complex probability amplitudes into actual probabilities. In one common form, it says that the squared modulus of a wave function that depends upon position is the probability density of measuring a particle as being at a given place. The integral of a wavefunction's squared modulus over all the system's degrees of freedom must be equal to 1, a condition called normalization. Since the wave function is complex-valued, only its relative phase and relative magnitude can be measured; its value does not, in isolation, tell anything about the magnitudes or directions of measurable observables. One has to apply quantum operators, whose eigenvalues correspond to sets of possible results of measurements, to the wave function ψ and calculate the statistical distributions for measurable quantities.

Wave functions can be functions of variables other than position, such as momentum. The information represented by a wave function that is dependent upon position can be converted into a wave function dependent upon momentum and vice versa, by means of a Fourier transform. Some particles, like electrons and photons, have nonzero spin, and the wave function for such particles includes spin as an intrinsic, discrete degree of freedom; other discrete variables can also be included, such as isospin. When a system has internal degrees of freedom, the wave function at each point in the continuous degrees of freedom (e.g., a point in space) assigns a complex number for each possible value of the discrete degrees of freedom (e.g., z-component of spin). These values are often displayed in a column matrix (e.g., a 2 × 1 column vector for a non-relativistic electron with spin 1⁄2).

According to the superposition principle of quantum mechanics, wave functions can be added together and multiplied by complex numbers to form new wave functions and form a Hilbert space. The inner product between two wave functions is a measure of the overlap between the corresponding physical states and is used in the foundational probabilistic interpretation of quantum mechanics, the Born rule, relating transition probabilities to inner products. The Schrödinger equation determines how wave functions evolve over time, and a wave function behaves qualitatively like other waves, such as water waves or waves on a string, because the Schrödinger equation is mathematically a type of wave equation. This explains the name "wave function", and gives rise to wave–particle duality. However, the wave function in quantum mechanics describes a kind of physical phenomenon, as of 2023 still open to different interpretations, which fundamentally differs from that of classic mechanical waves.^{[4][5][6][7][8][9][10]}

While a system of 3 bodies interacting gravitationally is chaotic, a system of 3 bodies interacting elastically is not.^{[clarification needed]}

Quantum entanglement

63 languages

• Article
• Talk

• Read
• Edit
• View history

Tools

From Wikipedia, the free encyclopedia

Quantum entanglement is the phenomenon of a group of particles being generated, interacting, or sharing spatial proximity in such a way that the quantum state of each particle of the group cannot be described independently of the state of the others, including when the particles are separated by a large distance. The topic of quantum entanglement is at the heart of the disparity between classical and quantum physics: entanglement is a primary feature of quantum mechanics not present in classical mechanics.^[1]

Measurements of physical properties such as position, momentum, spin, and polarization performed on entangled particles can, in some cases, be found to be perfectly correlated. For example, if a pair of entangled particles is generated such that their total spin is known to be zero, and one particle is found to have clockwise spin on a first axis, then the spin of the other particle, measured on the same axis, is found to be anticlockwise. However, this behavior gives rise to seemingly paradoxical effects: any measurement of a particle's properties results in an apparent and irreversible wave function collapse of that particle and changes the original quantum state. With entangled particles, such measurements affect the entangled system as a whole.

Such phenomena were the subject of a 1935 paper by Albert Einstein, Boris Podolsky, and Nathan Rosen,^[2] and several papers by Erwin Schrödinger shortly thereafter,^[3][4] describing what came to be known as the EPR paradox. Einstein and others considered such behavior impossible, as it violated the local realism view of causality (Einstein referring to it as "spooky action at a distance")^[5] and argued that the accepted formulation of quantum mechanics must therefore be incomplete.

Later, however, the counterintuitive predictions of quantum mechanics were verified^[6][7][8] in tests where polarization or spin of entangled particles were measured at separate locations, statistically violating Bell's inequality. In earlier tests, it could not be ruled out that the result at one point could have been subtly transmitted to the remote point, affecting the outcome at the second location.^[8] However, so-called "loophole-free" Bell tests have since been performed where the locations were sufficiently separated that communications at the speed of light would have taken longer—in one case, 10,000 times longer—than the interval between the measurements.^[7][6]

According to some interpretations of quantum mechanics, the effect of one measurement occurs instantly. Other interpretations which do not recognize wavefunction collapse dispute that there is any "effect" at all. However, all interpretations agree that entanglement produces correlation between the measurements, and that the mutual information between the entangled particles can be exploited, but that any transmission of information at faster-than-light speeds is impossible.^[9][10] Thus, despite popular thought to the contrary, quantum entanglement cannot be used for faster-than-light communication.^[11]

Quantum entanglement has been demonstrated experimentally with photons,^[12][13] electrons,^[14][15] top quarks,^[16] molecules^[17] and even small diamonds.^[18] The use of entanglement in communication, computation and quantum radar is an active area of research and development.

In 1900, Max Planck postulated the proportionality between the frequency ${\displaystyle 𝑓}$ of a photon and its energy ${\displaystyle 𝐸}$, ${\displaystyle 𝐸=ℎ𝑓}$,^[11][12] and in 1916 the corresponding relation between a photon's momentum ${\displaystyle 𝑝}$ and wavelength ${\displaystyle 𝜆}$, ${\displaystyle 𝜆=\frac{ℎ}{𝑝}}$,^[13] where ${\displaystyle ℎ}$ is the Planck constant. In 1923, De Broglie was the first to suggest that the relation ${\displaystyle 𝜆=\frac{ℎ}{𝑝}}$, now called the De Broglie relation, holds for massive particles, the chief clue being Lorentz invariance,^[14] and this can be viewed as the starting point for the modern development of quantum mechanics. The equations represent wave–particle duality for both massless and massive particles.

In the 1920s and 1930s, quantum mechanics was developed using calculus and linear algebra. Those who used the techniques of calculus included Louis de Broglie, Erwin Schrödinger, and others, developing "wave mechanics". Those who applied the methods of linear algebra included Werner Heisenberg, Max Born, and others, developing "matrix mechanics". Schrödinger subsequently showed that the two approaches were equivalent.^[15]

In 1926, Schrödinger published the famous wave equation now named after him, the Schrödinger equation. This equation was based on classical conservation of energy using quantum operators and the de Broglie relations and the solutions of the equation are the wave functions for the quantum system.^[16] However, no one was clear on how to interpret it.^[17] At first, Schrödinger and others thought that wave functions represent particles that are spread out with most of the particle being where the wave function is large.^[18] This was shown to be incompatible with the elastic scattering of a wave packet (representing a particle) off a target; it spreads out in all directions.^[1] While a scattered particle may scatter in any direction, it does not break up and take off in all directions. In 1926, Born provided the perspective of probability amplitude.^[1][2][19] This relates calculations of quantum mechanics directly to probabilistic experimental observations. It is accepted as part of the Copenhagen interpretation of quantum mechanics. There are many other interpretations of quantum mechanics. In 1927, Hartree and Fock made the first step in an attempt to solve the N-body wave function, and developed the self-consistency cycle: an iterative algorithm to approximate the solution. Now it is also known as the Hartree–Fock method.^[20] The Slater determinant and permanent (of a matrix) was part of the method, provided by John C. Slater.

Schrödinger did encounter an equation for the wave function that satisfied relativistic energy conservation before he published the non-relativistic one, but discarded it as it predicted negative probabilities and negative energies. In 1927, Klein, Gordon and Fock also found it, but incorporated the electromagnetic interaction and proved that it was Lorentz invariant. De Broglie also arrived at the same equation in 1928. This relativistic wave equation is now most commonly known as the Klein–Gordon equation.^[21]

In 1927, Pauli phenomenologically found a non-relativistic equation to describe spin-1/2 particles in electromagnetic fields, now called the Pauli equation.^[22] Pauli found the wave function was not described by a single complex function of space and time, but needed two complex numbers, which respectively correspond to the spin +1/2 and −1/2 states of the fermion. Soon after in 1928, Dirac found an equation from the first successful unification of special relativity and quantum mechanics applied to the electron, now called the Dirac equation. In this, the wave function is a spinor represented by four complex-valued components:^[20] two for the electron and two for the electron's antiparticle, the positron. In the non-relativistic limit, the Dirac wave function resembles the Pauli wave function for the electron. Later, other relativistic wave equations were found.

Weighing of the Heart[edit]

The heart of Hunefer weighed against the feather of Maat

In the Duat, the Egyptian underworld, the hearts of the dead were said to be weighed against her single "Feather of Maat", symbolically representing the concept of Maat, in the Hall of Two Truths. This is why hearts were left in Egyptian mummies while their other organs were removed, as the heart (called "ib") was seen as part of the Egyptian soul. If the heart was found to be lighter or equal in weight to the feather of Maat, the deceased had led a virtuous life and would go on to Aaru. Osiris came to be seen as the guardian of the gates of Aaru after he became part of the Egyptian pantheon and displaced Anubis in the Ogdoad tradition. A heart which was unworthy was devoured by the goddess Ammit and its owner condemned to remain in the Duat.^[58]

The weighing of the heart, as typically pictured on papyrus in the Book of the Dead, or in tomb scenes, shows Anubis overseeing the weighing and Ammit seated awaiting the results to consume those who failed. The image contains a balancing scale with an upright heart standing on one side and the Shu-feather standing on the other. Other traditions hold that Anubis brought the soul before the posthumous Osiris who performed the weighing. While the heart was weighed the deceased recited the 42 Negative Confessions as the Assessors of Maat looked on.^[58]

Assessors of Maat[edit]

Main article: Assessors of Maat

Some of the 42 Judges of Maat are visible, seated and in small size. Maat's feather of truth depicted in the bottom right corner. British Museum, London.

The Assessors of Maat are the 42 deities listed in the Papyrus of Nebseni,^[59] to whom the deceased make the Negative Confession in the Papyrus of Ani.^[60] They represent the forty-two united nomes of Egypt, and are called "the hidden Maati gods, who feed upon Maat during the years of their lives;" i.e., they are the righteous minor deities who deserve offerings.^[61] As the deceased follows the set formula of Negative Confessions, he addresses each god directly and mentions the nome of which the god is a patron, in order to emphasize the unity of the nomes of Egypt.^[59]

An animation of the figure-8 solution to the three-body problem over a single period T ≃ 6.3259^[13]

20 examples of periodic solutions to the three-body problem

Name of the deity	Identified with	Sin		Name of the deity	Identified with	Sin
1	"Far-Strider"	Heliopolis	falsehood	22	"Demolisher"	Xois	Transgressing
2	"Fire-Embracer"	Kheraha (Old Cairo?[13])	Robbery	23	"Disturber"	Weryt	Being hot-tempered
3	"Nosey One"	Hermopolis	Stealing	24	"Youth"	Heliopolitan nome	Unhearing of truth
4	"Swallower of Shades"	"The Cavern"	Murder	25	"Foreteller"	Wenes	Making disturbance
5	"Dangerous One"	Rosetau (Giza Plateau[14])	Stealing grain	26	"You of the Altar"	"the secret place"	Violence
6	"Double Lion"	"The sky"	Prolonging offerings	27	"Face Behind Him"	"Cavern of wrong"	copulating with a boy
7	"Fiery Eyes"	Letopolis	Stealing Gods property	28	"Hot-Foot"	"The dusk"	Transgression
8	"Flame"	"Came forth backwards"	Lying	29	"You of the Darkness"	"The darkness"	Quarrelling
9	"Bone Breaker"	Heracleopolis	Taking food	30	"Bringer of Your Offerings"	Sais	Unduly active
10	"Green of Flame"	Memphis	Cursing	31	"Owner of Faces"	Nedjefet (13th / 14th Upper Egyptian nome)	Impatience
11	"You of the Cavern"	"The West"	Adultery	32	"Accuser"	Wetjenet (in Punt[15])	damaging a god's image
12	"White of Teeth"	Faiyum	Causing tears	33	"Owner of Horns"	Asyut	Volubility of speech
13	Shezmu	"The shambles"	Killing a sacred bull	34	Nefertem	Memphis	Wrongdoing
14	"Eater of Entrails"	"House of Thirty"	Stealing land	35	Temsep	Busiris	Conjuration against the king
15	"Lord of Truth"	Maaty	Eavesdropping	36	"You Who Acted Willfully"	Tjebu	Stopping water flow
16	"Wanderer"	Bubastis	Complaints	37	"Water-Smiter"	"The abyss"	Being loud voiced
17	"Pale One"	Heliopolis	Being angry	38	"Commander of Mankind"	"Your house"	Reviling God
18	"Doubly Evil"	Andjet	Adultery	39	Nehebkau	The Harpoon Nome (7th / 8th Lower Egyptian nome[16])	Doing ... ?
19	"Wememty-Snake"	"Place of execution"	Adultery	40	"Bestower of Powers"	"The city"	Making distinctions For self
20	"See Whom You Bring"	"House of Min"	Polluting the body	41	"Serpent With Raised Head"	"The cavern"	dishonest wealth
21	"Over the Old One"	Imau	Terrorizing	42	"Serpent Who Brings and Gives"	"The silent land"	Blasphemy